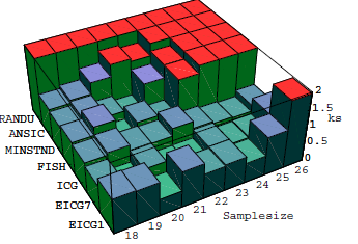
SFB funding period 1 (2014-2017)

This project is about techniques relevant to the theory of uniform distribution modulo 1 (u.d.mod 1) of sequences and its applications in the field of random number generation and QMC methods. There is also a tie of this project to applied cryptography.

We will contribute (i) to the question how well a given (finite) sequence is u.d.mod 1, by studying and developing appropriate figures of merit and investigating their relation (keywords: discrepancy, diaphony, spectral test, inequality of Erdös-Turán-Koksma, inequality of Le Veque), (ii) to new construction methods for so-called low discrepancy sequences (keyword: $b$-adic arithmetics, lattice methods), and (iii) to applied cryptography (keywords: nonlinearity of Boolean functions, bit diffusion).